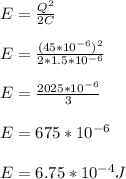Answer:
a) Energy stored in the capacitor,
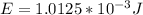
b) Q = 45 µC
c) C' = 1.5 μF
d)

Step-by-step explanation:
Capacitance, C = 1 µF
Charge on the plates, Q = 45 µC
a) Energy stored in the capacitor is given by the formula:

b) The charge on the plates of the capacitor will not change
It will still remains, Q = 45 µC
c) Electric field is non zero over (1-1/3) = 2/3 of d
From the relation V = Ed,
The voltage has changed by a factor of 2/3
Since the capacitance is given as C = Q/V
The new capacitance with the conductor in place, C' = (3/2) C
C' = (3/2) * 1μF
C' = 1.5 μF
d) Energy stored in the capacitor with the conductor in place