Question

The accounting department analyzes the variance of the weekly unit costs reported by two production departments. A sample of 16 cost reports for each of the two departments shows cost variances of 2.5 and 5.5, respectively. Is this sample sufficient to conclude that the two production departments differ in terms of unit cost variance? Use = .10. State the null and alternative hypotheses.

Answer 1

`F=(s^2_2)/(s^2_1)=(5.5)/(2.5)=2.2`

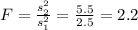
Now we can calculate the p value but first we need to calculate the degrees of freedom for the statistic. For the numerator we have
`n_2 -1 =16-1=15` and for the denominator we have
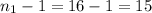`n_1 -1 =16-1=15` and the F statistic have 15 degrees of freedom for the numerator and 15 for the denominator. And the P value is given by:

`p_v =2*P(F_(15,15)>2.2)=0.138`

For this case the p value is highert than the significance level so we haev enough evidence to FAIL to reject the null hypothesis and we can conclude that the true deviations are not significantly different

Explanation:

Information given

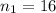`n_1 = 16` represent the sampe size 1

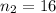`n_2 =16` represent the sample 2

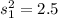`s^2_1 = 2.5` represent the sample deviation for 1

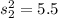`s^2_2 = 5.5` represent the sample variance for 2

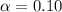`\alpha=0.10` represent the significance level provided

The statistic is given by:

`F=(s^2_2)/(s^2_1)`

Hypothesis to test

We want to test if the variations in terms of the variance are equal, so the system of hypothesis are:

H0:
`\sigma^2_1 = \sigma^2_2`

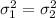
H1:
`\sigma^2_1 \\eq \sigma^2_2`

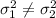
The statistic is given by:

`F=(s^2_2)/(s^2_1)=(5.5)/(2.5)=2.2`

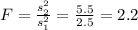
Now we can calculate the p value but first we need to calculate the degrees of freedom for the statistic. For the numerator we have
`n_2 -1 =16-1=15` and for the denominator we have
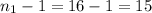`n_1 -1 =16-1=15` and the F statistic have 15 degrees of freedom for the numerator and 15 for the denominator. And the P value is given by:

`p_v =2*P(F_(15,15)>2.2)=0.138`

For this case the p value is highert than the significance level so we haev enough evidence to FAIL to reject the null hypothesis and we can conclude that the true deviations are not significantly different