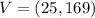Question

Determine the vertex of (f)=-×^2+50×-456

Answer 1

`V_x = -(50)/(2*(-1)) =25`

And for the coordinate on y we can use the function like this:

`V_y = -(25)^2 +50*25 -456 =169`

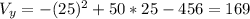
Then the vertex would be
`V= (25,169)`

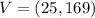
Explanation:

For this problem we have the following function given:

`f(x) = -x^2 +50x -456`

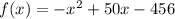
That represent a quadratic function and the general form is given by:

`f(x)= ax^2 +bx +c`

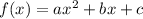
For this problem a =-1 , b= 50 , c=-456 and we can find the corrdinate of the vertex in x with this formula:

`V_x =-(b)/(2a)`

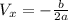
And replacing we got:

`V_x = -(50)/(2*(-1)) =25`

And for the coordinate on y we can use the function like this:

`V_y = -(25)^2 +50*25 -456 =169`

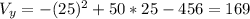
Then the vertex would be
`V= (25,169)`