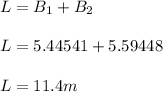Question

A new landowner has a triangular piece of flat land she wishes to fence. Starting at the first corner, she measures the first side to be 5.7 m long and is directed 0.3 radians north of east. From the second corner, the second side is 9 m long and is directed 0.9 radians west of north. What is the length of the third side of the fence?

Answer 1

The length of the third side of fence is 11.4 m

Explanation:

Solution:-

- We are to mow a triangular piece of land. We are given the description of motion and the orientation of land-mower while fencing.

- From one corner of the triangular land, the land-mower travels H1 = 5.7 m at θ1 = 0.3 radians north of east. We will use trigonometric ratios to determine the amount traveled ( B1 ) in the east direction.

`cos ( theta_1 ) = (B_1)/(H_1)`

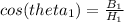
Where,

B1: Is the base length of the right angle triangle

H1: Hypotenuse of the right angle triangle

Therefore,

`B_1 = H_1*cos ( theta_1 )\\\\B_1 = 5.7*cos ( 0.3 )\\\\B_1 = 5.44541 m`

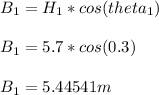
- Similarly, from the other corner of the triangular land. The land-mower moves a lateral distance of H2 = 9m and directed θ2 = 0.9 radians north of west. We will use trigonometric ratios to determine the amount traveled ( B2 ) in the west direction.

`cos ( theta_2 ) = (B_2)/(H_2) \\`

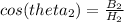
Where,

B2: Is the base length of the right angle triangle

H2: Hypotenuse of the right angle triangle

Therefore,

`B_2 = H_2*cos ( theta_2 )\\\\B_2 = 9*cos(0.9)\\\\B_2 = 5.59448 m`

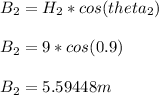
- The total length of the third side of the fence would be the sum of bases of the two right angles formed by the land-mower motion at each corner.

`L = B_1 + B_2\\\\L = 5.44541 + 5.59448\\\\L = 11.4 m`