Answer:
a) v_correcaminos = 22.95 m / s , b) x = 512.4 m ,
c) v = (45.83 i ^ -109.56 j ^) m / s
Step-by-step explanation:
We can solve this exercise using the kinematics equations
a) Let's find the time or the coyote takes to reach the cliff, let's start by finding the speed on the cliff
v² = v₀² + 2 a x
they tell us that the coyote starts from rest v₀ = 0 and its acceleration is a=15 m / s²
v = √ (2 15 70)
v = 45.83 m / s
with this value calculate the time it takes to arrive
v = v₀ + a t
t = v / a
t = 45.83 / 15
t = 3.05 s
having the distance to the cliff and the time, we can find the constant speed of the roadrunner
v_ roadrunner = x / t
v_correcaminos = 70 / 3,05
v_correcaminos = 22.95 m / s
b) if the coyote leaves the cliff with the horizontal velocity v₀ₓ = 45.83 m / s, they ask how far it reaches.
Let's start by looking for the time to reach the cliff floor
y = y₀ +
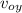 t - ½ g t²
t - ½ g t²
in this case y = 0 and the height of the cliff is y₀ = 100 m
0 = 100 + 45.83 t - ½ 9.8 t²
t² - 9,353 t - 20,408 = 0
we solve the quadratic equation
t = [9,353 ±√ (9,353² + 4 20,408)] / 2
t = [9,353 ± 13] / 2
t₁ = 11.18 s
t₂ = -1.8 s
Since time must be a positive quantity, the answer is t = 11.18 s
we calculate the horizontal distance traveled
x = v₀ₓ t
x = 45.83 11.18
x = 512.4 m
c) speed when it hits the ground
vₓ = v₀ₓ = 45.83 m / s
we look for vertical speed
v_{y} =
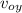 - gt
- gt
v_{y} = 0 - 9.8 11.18
v_{y} = - 109.56 m / s
v = (45.83 i ^ -109.56 j ^) m / s