Answer:
Yes
Explanation:
The Mean Value Theorem states that if f(x) is defined and continuous on the interval [a,b] and differentiable on (a,b), then there is at least one number c in the interval (a,b) (that is a < c < b) such that

Given
![f(x)=x^3+x-9$ in [0,2]](https://img.qammunity.org/2021/formulas/mathematics/college/q5wifmwqe8p84blh5io55zgc1vnjbvhog1.png)
f(x) is defined, continuous and differentiable.
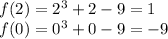
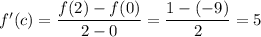

Therefore:
![f'(c)=3c^2+1=5\\3c^2=5-1\\3c^2=4\\c^2=(4)/(3) \\c=\sqrt{(4)/(3)} =1.15 \in [0,2]](https://img.qammunity.org/2021/formulas/mathematics/college/6o7s0i0fqgfew4psa44ej9uj25wonf3wtc.png)
Since c is in the given interval, the function satisfy the hypotheses of the Mean Value Theorem on the given interval.