Question

A proton moves at a speed 1.4 × 10^7 m/s perpendicular to a magnetic field. The field causes the proton to travel in a circular path of radius 0.85 m. What is the field strength?

Answer 1

0.17T

Step-by-step explanation:

When a charged particle moves into a magnetic field perpendicularly, it experiences a magnetic force
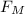`F_(M)` which is perpendicular to the magnetic field and direction of the velocity. This motion is circular and hence there is a balance between the centripetal force
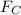`F_(C)` and the magnetic force. i.e

`F_(C)` =
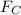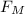`F_(M)` --------------(i)

But;

`F_(C)` =
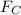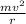`(mv^2)/(r)` [m = mass of the particle, r = radius of the path, v = velocity of the charge]

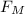`F_(M)` = qvB [q = charge on the particle, B = magnetic field strength, v = velocity of the charge ]

Substitute these into equation (i) as follows;

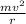`(mv^2)/(r)` = qvB

Make B subject of the formula;

B =
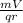`(mV)/(qr)` ---------------(ii)

Known constants

m = 1.67 x 10⁻²⁷kg

q = 1.6 x 10⁻¹⁹C

From the question;

v = 1.4 x 10⁷m/s

r = 0.85m

Substitute these values into equation(ii) as follows;

B =
`(1.67 * 10 ^(-27) * 1.4 * 10^(7))/(1.6 * 10^(-19) * 0.85)`

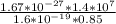
B = 0.17T

Therefore, the magnetic field strength is 0.17T