Question

6.1.3

What requirements are necessary for a normal probability distribution to be a standard normal probability distribution?

Answer 1

The requirements that are necessary for a normal probability distribution to be a standard normal probability distribution are µ = 0 and σ = 1.

Explanation:

A normal-distribution is an accurate symmetric-distribution of experimental data-values.

If we create a histogram on data-values that are normally distributed, the figure of columns form a symmetrical bell shape.

If X
`\sim` N (µ, σ²), then
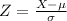`Z=(X-\mu)/(\sigma)`, is a standard normal variate with mean, E (Z) = 0 and Var (Z) = 1. That is, Z
`\sim` N (0, 1).

The distribution of these z-variates is known as the standard normal distribution.

Thus, the requirements that are necessary for a normal probability distribution to be a standard normal probability distribution are µ = 0 and σ = 1.