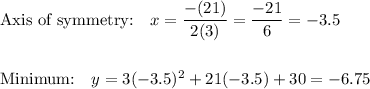Answer: see below
Explanation:
Given ax² + bx + c = 0
If a > 0 (positive), then parabola opens UP
If a < 0 (negative), then parabola opens (DOWN)
Axis of Symmetry:
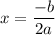
Max/Min: Plug axis of symmetry into the given equation and solve for y.
1) y = x² + 11x + 24
a=1 b=11
a> 0 so parabola opens UP
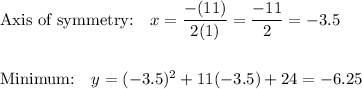
2) y = -x² - 6x - 8
a=-1 b=-6
a< 0 so parabola opens DOWN

3) y = x² - 2x + 3
a=1 b=-2
a> 0 so parabola opens UP

4) y = x² + 4x + 4
a=1 b=4
a> 0 so parabola opens UP

5) y = 3x² + 21x + 30
a=3 b=21
a> 0 so parabola opens UP