Answer:
The height of the tree is 35.6 ft
Explanation:
For this equation, we are going to use tan. We are using tan because we are given the side length for the adjacent side and we are looking for the side length of the opposite side which is also known as our tree's height.
Let's set up an equation.
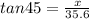
Since we do not know what the value of x is, then we are going to multiply 35.6 on both sides of the equation so we can get x by itself.
(35.6)tan45 = x/35.6 (35.6)
x = 35.6(tan45)
Now, we are going to multiply 35.6 by tan45. You can use a calculator when multiplying this one.
x = 35.6
The value of x is 35.6 which is also the height of our tree.