Answer:
Height of tree is
 15 m.
15 m.
Explanation:
Given that student is 20 m away from the foot of tree.
and table is 1.5 m above the ground.
The angle of elevation is: 34°28'
Please refer to the attached image. The given situation can be mapped to a right angled triangle as shown in the image.
AB = CP = 20 m
CA = PB = 1.5 m
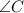 = 34°28' = 34.46°
= 34°28' = 34.46°
To find TB = ?
we can use trigonometric function tangent to find TP in right angled
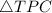

Now, adding PB to TP will give us the height of tree, TB
Now, height of tree TB = TP + PB
TB = 13.72 + 1.5 = 15.22
 15 m
15 m