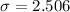Answer:
Expected value or mean =

Variance =
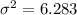
Standard deviation =
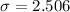
Explanation:
We are given the following information:
x | P(x)
6 | 0.64
8 | 0.14
9 | 0.14
15 | 0.08
The expected value or mean is given by
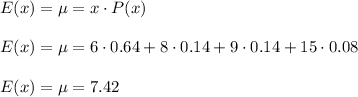
The variance is given by

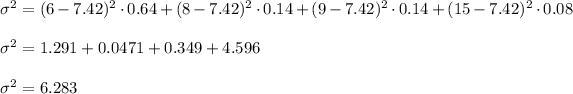
The standard deviation is given by
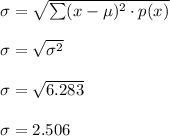
Therefore,
Expected value or mean =

Variance =
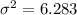
Standard deviation =