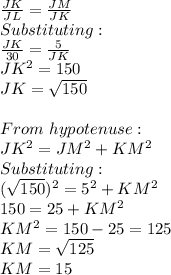Answer:
KM = 15
Explanation:
From the diagram, ΔJKL and ΔJKM are similar to each other because they share the same angle J and they are both right angle triangle. Therefore they are similar by AA property.
Since JL=25 and JM=5, JM = JL + JM= 25 + 5 = 30
Since ΔJKL and ΔJKM are similar to each other, therefore: