Question

A 65.0 kg skier is moving at 6.85 m/s on a frictionless, horizontal snow-covered plateau when she encounters a rough patch 4.00 m long. The coefficient of kinetic friction between this patch and her skis is 0.330. After crossing the rough patch and returning to friction-free snow, she skis down an icy, frictionless hill 2.60 m high. Part A How fast is the skier moving when she gets to the bottom of the hill

Answer 1

v = 4.58 m/s

Step-by-step explanation:

In order to calculate the speed of the skier when she gets the bottom of the hill, you have to calculate the speed of the skier when she crosses the rough patch.

To calculate the velocity at the final of the rough patch you take into account that the work done by the friction surface is equal to the change in the kinetic energy of the skier:

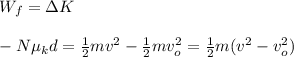`W_f=\Delta K\\\\-N\mu_kd=(1)/(2)mv^2-(1)/(2)mv_o^2=(1)/(2)m(v^2-v_o^2)` (1)

Where the minus sign means that the work is against the motion of the skier.

Wf: friction force

m: mass of the skier = 65.0kg

N: normal force = mg

g: gravitational acceleration = 9.8m/s^2

d: distance of the rough patch = 4.00m

v: speed at the end of the rough patch = ?

vo: initial speed of the skier = 6.85m/s

μk: coefficient of kinetic friction = 0.330

You replace the expression for the normal force in the equation (1), and solve for v:

`-mg\mu_kd=(1)/(2)m(v^2-v_o^2)\\\\-g\mu_kd=(1)/(2)(v^2-v_o^2)\\\\v=√(-2g\mu_kd+v_o^2)\\\\v=√(-2(9.8m/s^2)(0.330)(4.00m)+(6.85m/s)^2)=8.53(m)/(s)=4.58(m)/(s)`

Then, the speed fot he skier at the bottom of the hill is 4.58m/s