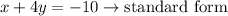Explanation:
The point-slope form of an equation of a line:

where the slope m is:

We have the points (2, -3) and (-6, -1).
Substitute:
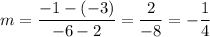
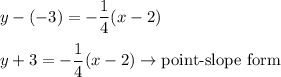
The slope-intercept form of an equation of a line:


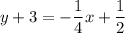 subtract 3 from both sides
subtract 3 from both sides
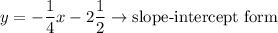
The standard form of an equation of a line:
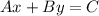
 multiply both sides by 4
multiply both sides by 4
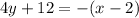
 subtract 12 from both sides
subtract 12 from both sides
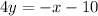 add x to both sides
add x to both sides