Answer:
Speed = 20 m/sec at 75 deg South of East = 20 m/sec at 15 deg East of South
Step-by-step explanation:
given data
truck moves = 25 m/s toward the east.
throws a baseball = 28 m/s southwest
solution
first we take here Speed of truck w.r.to ground i.e. V(p/g) = 25 m/sec toward the east so we can say
V(p/g) = (25 i) m/sec ........................1
and
Speed of baseball w.r.t. pickup i.e. V(b/p) = 28 m/sec toward the South West and we know that south west direction is in third quadrant
and here both component (x and y) are negative
So that we can say it
V(b/p) = -28 × cos(45) i - 28 × sin(45) j = -19.8 i - 19.8 j
and
now we use here relative motion velocity for ball w.r.t ground
V(b/g) = V(b/p) + V(p/g ) ..........................2
put here value and we get
V(b/g) = (-19.8 i - 19.8 j) + 25 i = 5.2 i - 19.8 j
so
Magnitude of that velocity
| V(b/g) | =
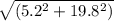
| V(b/g) | = 20.47 m/sec
so that Direction will be here
Direction = arctan (19.8 ÷ 5.2)
Direction = 75.3° South of East
so that
Speed = 20.47 m/sec at 75.3 deg South of East
and 2 significant
Speed = 20 m/sec at 75 deg South of East = 20 m/sec at 15 deg East of South