Answer:
![A(t)=500C_(in)(t)+[40-500C_(in)(t)]\cdot e^{-(t)/(100)}](https://img.qammunity.org/2021/formulas/mathematics/college/7myc5nbq27j714pqfiqks50wgd81yl2sky.png)
Step-by-step explanation:
Volume of water in the Tank =500 gallons
Let A(t) be the amount of salt in the tank at time t.
Initially, the tank contains 40 lbs of salt, therefore:
A(0)=40 lbs
Rate of change of the amount of Salt in the Tank
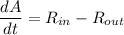
Rate In=(concentration of salt in inflow)(input rate of brine)
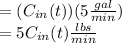
Rate Out=(concentration of salt in outflow)(output rate of brine)
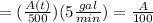
Therefore:
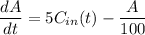
We then solve the resulting differential equation by separation of variables.
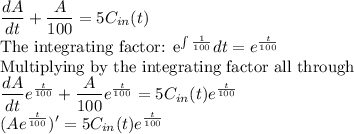
Taking the integral of both sides
![\int(Ae^{(t)/(100)})'=\int [5C_(in)(t)e^{(t)/(100)}]dt\\Ae^{(t)/(100)}=5*100C_(in)(t)e^{(t)/(100)}+C, $(C a constant of integration)\\Ae^{(t)/(100)}=500C_(in)(t)e^{(t)/(100)}+C\\$Divide all through by e^{(t)/(100)}\\A(t)=500C_(in)(t)+Ce^{-(t)/(100)}](https://img.qammunity.org/2021/formulas/mathematics/college/96rnt1hvbqiby084442qsmrvy6ok2ffpcb.png)
Recall that when t=0, A(t)=40 lbs (our initial condition)
![A(t)=500C_(in)(t)+Ce^{-(t)/(100)}\\40=500C_(in)(t)+Ce^{-(0)/(100)}\\C=40-500C_(in)(t)\\$Therefore, the amount of salt in the tank at any time t is:\\\\A(t)=500C_(in)(t)+[40-500C_(in)(t)]\cdot e^{-(t)/(100)}](https://img.qammunity.org/2021/formulas/mathematics/college/jso7b1mvap9ihd7q9ygmbz7kizj2zpz46w.png)