Question

An aluminum bar 600mm long with diameter 40mm, has a hole drilled in the center of the bsr.The hole is 30mm in diameter and is 100mm long. If the modulus of elasticity for the aluminum is 85GN/m2, calculate the total contraction on the bar due to a compressive load of 180KN

Answer 1

1.228 x
`10^(-6)` mm

Step-by-step explanation:

diameter of aluminium bar D = 40 mm

radius of aluminium bar R = D/2 = 40/2 = 20 mm

diameter of hole d = 30 mm

radius of the hole r = d/2 = 30/2 = 15 mm

compressive Load F = 180 kN = 180 x
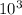`10^(3)` N

modulus of elasticity E = 85 GN/m^2 = 85 x
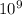`10^(9)` Pa

length of bar L = 600 mm

length of hole = 100 mm

true length of bar = 600 - 100 = 500 mm

area of the bar A =
`\pi r^(2)` = 3.142 x
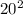`20^(2)` = 1256.8 mm^2

area of hole a =
`\pi (R^(2) - r^(2))` =
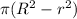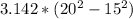`3.142* (20^(2) - 15^(2))` = 549.85 mm^2

Total contraction of the bar =
`(F*L)/(AE) + (Fl)/(aE)`

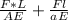
total contraction =
`(F)/(E) * ((L)/(A) +(l)/(a))`

==>
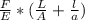
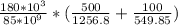`(180*10^(3))/(85*10^(9)) *( (500)/(1256.8) + (100)/(549.85))` = 1.228 x
`10^(-6)` mm