Answer:
Explained below.
Explanation:
Consider the series is a set of first 6 natural numbers.
Sum of first n terms is:
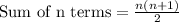
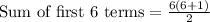
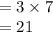
Consider the series is an arithmetic sequence.
Sum of first n terms is:
![S_(n)=(n)/(2) [2a+(n-1)d]](https://img.qammunity.org/2021/formulas/mathematics/high-school/grms7caveq71lrtsb75nschndxhcno0oev.png)
Here,
a = first term
d = common difference
Consider the series is an geometric sequence.
Sum of first n terms is:
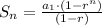
Here,
a₁ = first term
r = common ratio