Question Correction
The table represents an exponential function. What is the multiplicative rate of change of the function?
(A)1/3 (B)2/3 (C)2 (D)9
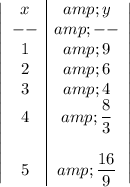
Answer:
(B)

Explanation:
An exponential function is a function of the form
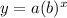
where a is the initial value and b is the multiplicative rate of change
When x=2, y=6, we have:
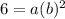
When x=3, y=4, we have:
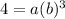
Dividing the two equations:
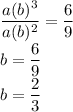
The multiplicative rate of change, b is
 .
.
The correct option is B.