Question

Find the focus and the directrix of the parabola with the equation y = 1∕4(x – 6)2+ 5

Question 16 options:

A)

Focus = (5,–6), directrix is y = –7

B)

Focus = (6,6), directrix is y = 4

C)

Focus = (6,–6), directrix is y = –4

D)

Focus = (–5,6), directrix is y = –4

Answer 1

B)

Focus = (6,6), directrix is y = 4

Explanation:

A parabola is the locus of a point that is equidistant from a fixed line (known as directrix) and a fixed point (known as focus).

The equation of a parabola in vertex form is given as:

`y=a(x-h)^2+k`

It can be further simplified to:

`(x-h)^2=4p(y-k)`

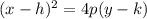
The directrix of this parabola is given at line y = k - p and the focus is at (h, k + p)

Given that:

`y = (1)/(4)(x-6)^2+ 5 \\(y-5)=(1)/(4)(x-6)^2\\(x-6)^2=4(y-5)`

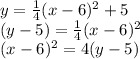
Comparing (x-6)² = 4(y-5) with (x-h)² = 4p(y-k), It can be gotten that h = 6, p =1 and k = 5

The directrix of this parabola is given at line y = k - p = 5 - 1 = 4 and the focus is at (h, k + p) = (6, 5+1) = (6, 6)

Focus = (6,6), directrix is y = 4