Question

The U.S. Department of Agriculture (USDA) uses sample surveys to obtain important economic estimates. One USDA pilot study estimated the price received by farmers for corn sold in January from a sample of 20 farms. The mean price was reported as $3.64 per bushel with a standard deviation of $0.0835 per bushel. Give a 95% confidence interval for the mean price received by farmers for corn sold in January.

Answer 1

{$3.60; $3.68}

Explanation:

The confidence interval for a sample of size 'n', with mean price 'X' and standard deviation 's' is determined by:

`X\pm z*(s)/(\sqrt n)`

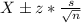
The z-score for a 95% confidence interval is 1.96.

Applying the given data, the lower and upper bounds of the confidence interval are:

`3.64\pm 1.96*(0.0835)/(\sqrt 20) \\L=\$3.60\\U=\$3.68`

The confidence interval for the mean price received by farmers for corn sold in January is:

CI : {$3.60; $3.68}