Answer:
Total volume of the figure = 1067 cm³
Explanation:
Volume of the composite figure = Volume of the rectangular prism + Volume of the square pyramid
Volume of the rectangular prism = Length × Width × Height
= 10 × 10 × 7
= 700 cm³
Volume of the square pyramid =
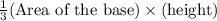
=
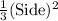 × height
× height
=

= 366.67 cm³
Total volume of the composite figure = 700 + 366.67 = 1066.67 cm³
≈ 1067 cm³