Question

The curvature of a plane parametric curve x = f(t), y = g(t) is $ \kappa = \dfrac{|\dot{x} \ddot{y} - \dot{y} \ddot{x}|}{[\dot{x}^2 + \dot{y}^2]^{3/2}}$ where the dots indicate derivatives with respect to t. Use the above formula to find the curvature. x = 6et cos(t), y = 6et sin(t)

Answer 1

The curvature is modelled by
`\kappa = (e^(-t))/(6√(2))`.

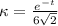Explanation:

The equation of the curvature is:

`\kappa = \frac{|\dot {x}\cdot \ddot {y}-\dot{y}\cdot \ddot{x}|}{[\dot{x}^(2)+\dot{y}^(2)]^{(3)/(2) }}`

The parametric componentes of the curve are:

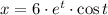`x = 6\cdot e^(t) \cdot \cos t` and
`y = 6\cdot e^(t)\cdot \sin t`

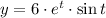
The first and second derivative associated to each component are determined by differentiation rules:

First derivative

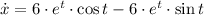`\dot{x} = 6\cdot e^(t)\cdot \cos t - 6\cdot e^(t)\cdot \sin t` and
`\dot {y} = 6\cdot e^(t)\cdot \sin t + 6\cdot e^(t) \cdot \cos t`

`\dot x = 6\cdot e^(t) \cdot (\cos t - \sin t)` and
`\dot {y} = 6\cdot e^(t)\cdot (\sin t + \cos t)`

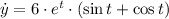
Second derivative

`\ddot{x} = 6\cdot e^(t)\cdot (\cos t-\sin t)+6\cdot e^(t) \cdot (-\sin t -\cos t)`

`\ddot x = -12\cdot e^(t)\cdot \sin t`

`\ddot {y} = 6\cdot e^(t)\cdot (\sin t + \cos t) + 6\cdot e^(t)\cdot (\cos t - \sin t)`

`\ddot{y} = 12\cdot e^(t)\cdot \cos t`

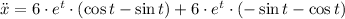
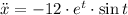
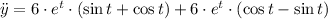
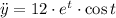
Now, each term is replaced in the the curvature equation:

`\kappa = \frac{\left\{\left[6\cdot e^(t)\cdot (\cos t - \sin t)\right]^(2)+\right[6\cdot e^(t)\cdot (\sin t + \cos t)\left]^(2)\right\}^{(3)/(2)}} }`

And the resulting expression is simplified by algebraic and trigonometric means:

`\kappa = \frac{72\cdot e^(2\cdot t)\cdot \cos^(2)t-72\cdot e^(2\cdot t)\cdot \sin t\cdot \cos t + 72\cdot e^(2\cdot t)\cdot \sin^(2)t+72\cdot e^(2\cdot t)\cdot \sin t \cdot \cos t}{[36\cdot e^(2\cdot t)\cdot (\cos^(2)t -2\cdot \cos t \cdot \sin t +\sin^(2)t)+36\cdot e^(2\cdot t)\cdot (\sin^(2)t+2\cdot \cos t \cdot \sin t +\cos^(2) t)]^{(3)/(2) }}`

`\kappa = \frac{72\cdot e^(2\cdot t)}{[72\cdot e^(2\cdot t)]^{(3)/(2) } }`

`\kappa = [72\cdot e^(2\cdot t)]^{-(1)/(2) }`

`\kappa = 72^{-(1)/(2) }\cdot e^(-t)`

`\kappa = (e^(-t))/(6√(2))`

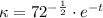
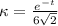
The curvature is modelled by
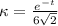`\kappa = (e^(-t))/(6√(2))`.