Question

Two basketball players, Jerry and Henry, want to find out who has the greater height when compared to each of their teams. Jerry has a height of 72.5 inches, and his team has a mean height of 75.5 inches and a standard deviation of 2 inches. Henry has a height of 73 inches, and his team has a mean of 75 inches and a standard deviation of 2.5 inches. Who has the greater height when compared to each of their teams?

Answer 1

Due to the higher z-score, Henry has the greater height when compared to each of their teams

Explanation:

Z-score:

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Who has the greater height when compared to each of their teams?

Whoever has the higher z-score.

Jerry:

Jerry has a height of 72.5 inches, and his team has a mean height of 75.5 inches and a standard deviation of 2 inches. So
`X = 72.5, \mu = 75.5, \sigma = 2`

`Z = (X - \mu)/(\sigma)`

`Z = (72.5 - 75.5)/(2)`

`Z = -1.5`

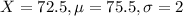
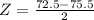
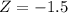
Henry:

Henry has a height of 73 inches, and his team has a mean of 75 inches and a standard deviation of 2.5 inches. So
`X = 73, \mu = 75, \sigma = 2.5`

`Z = (X - \mu)/(\sigma)`

`Z = (73 - 75)/(2.5)`

`Z = -0.8`

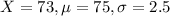
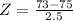
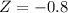
Due to the higher z-score, Henry has the greater height when compared to each of their teams