Answer:
t= 1,56 s , x= 124,8 m , v = (80 i^ - 15,288 j ) m/s
Step-by-step explanation:
Este es un ejercicio de lanzamiento proyectiles, comencemos por encontrar el tiempo que tarda en llegar al piso
y = y₀ +
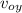 t – ½ g t²
t – ½ g t²
en este caso la altura inicial es y₀= 12 m y llega a y=0 , como es lanzado horizontalmente la velocidad vertical es cero (v_{oy}=0)
0 = y₀ – ½ g t²
t= √ (2 y₀/g)
calculemos
t= √ ( 2 12 / 9,8)
t= 1,56 s
El alcance del proyectil es la distancia horizontal recorrida
x = v₀ₓ t
x = 80 1,56
x= 124,8 m
La velocidad de impacto cuando toca el suelo
vx = v₀ₓ = 80 ms
 = v_{oy} – gt
= v_{oy} – gt
v_{y} = - 9,8 1,56
v_{y} = - 15,288 m/s
la velocidad es
v = (80 i^ - 15,288 j ) m/s
Traducttion
This is a projectile launching exercise, let's start by finding the time it takes to reach the ground
y = y₀ + v_{oy} t - ½ g t²
in this case the initial height is i = 12 m and it reaches y = 0, as it is thrown horizontally the vertical speed is zero (
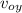 = 0)
= 0)
0 =y₀I - ½ g t²
t = √ (2y₀ / g)
let's calculate
t = √ (2 12 / 9.8)
t = 1.56 s
Projectile range is the horizontal distance traveled
x = v₀ₓ t
x = 80 1.56
x = 124.8 m
Impact speed when it hits the ground
vₓ = v₀ₓ = 80 ms
v_{y} = v_{oy} - gt
v_{y} = - 9.8 1.56
v_{y} = - 15,288 m / s
the speed is
v = (80 i ^ - 15,288 j) m / s