Question

The number of traffic accidents that occur on a particular stretch of road during a month follows a Poisson distribution with a mean of 8.1. Find the probability that the next two months will both result in three accidents each occuring in this stretch of road

Answer 1

P = 0.00072

Explanation:

If the number of traffic accidents that occurs follows a Poisson distribution, the probability that x traffic accidents occurs is:

`P(x)=(e^(-m)(m^x))/(x!)`

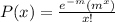
Where m is the mean. So replacing m by 8.1 accidents/monts, we get:

`P(x)=(e^(-8.1)(8.1^x))/(x!)`

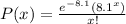
So, the probability that the next two months will both result in three accidents each occurring in this stretch of road is calculated as:

`P(3)*P(3)=(e^(-8.1)(8.1^3))/(3!)*(e^(-8.1)(8.1^3))/(3!)\\P(3)*P(3)=0.00072`