Answer:
Option D
Explanation:
We are given the following equations -
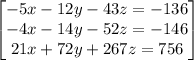
It would be best to solve this equation in matrix form. Write down the coefficients of each terms, and reduce to " row echelon form " -
 First, I swapped the first and third rows.
First, I swapped the first and third rows.
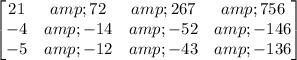 Leading coefficient of row 2 canceled.
Leading coefficient of row 2 canceled.
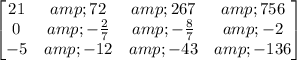 The start value of row 3 was canceled.
The start value of row 3 was canceled.
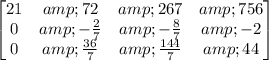 Matrix rows 2 and 3 were swapped.
Matrix rows 2 and 3 were swapped.
 Leading coefficient in row 3 was canceled.
Leading coefficient in row 3 was canceled.
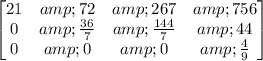
And at this point, I came to the conclusion that this system of equations had no solutions, considering it reduced to this -
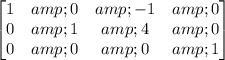
The positioning of the zeros indicated that there was no solution!
Hope that helps!