Question

Two stationary positive point charges, charge 1 of magnitude 4.00 nC and charge 2 of magnitude 1.55 nC , are separated by a distance of 34.0 cm . An electron is released from rest at the point midway between the two charges, and it moves along the line connecting the two charges.

Required:
What is the speed of the electron when it is 10.0 cm from the +3.00-nC charge?

Answer 1

v = 4.31*10^6 m/s

Step-by-step explanation:

In order to calculate the speed of the electron, you first calculate the acceleration of the electron, generated by the electric force.

You use the following formula:

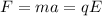`F=ma=qE` (1)

m: mass of the electron = 9.1*10-31kg

a: acceleration of the electron = ?

q: charge of the electron = 1.6*10^-19C

E: electric field

Then, it is necessary to calculate the electric field, which is given by:

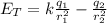`E_T=k(q_1)/(r_1^2)-(q_2)/(r_2^2)` (2)

The electric field is calculated at the point midway of the two charges q1 and q2.

The minus sing means that the electric force are opposite in such a point.

q1: charge 1 = 4.00nC = 4.00*10^-9 C

q2: charge 2 = 1.55nC = 1.55*10^-19 C

r1: distance to the first particle = 34.0cm/2 = 17.0cm = 0.17m

r2: distance to the second charge = 0.17m

Then, the electric field is:

`E_T=(k)/(r^2)(q_1-q_2)=((8.98*10^9Nm^2/C^2))/((0.17m)^2)(4.00*10^(-9)C-1.55*10^(-9)C)\\\\E_T=761.28(N)/(C)`

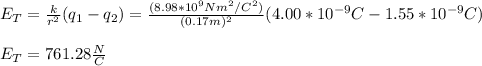
The electron moves toward the first charge, because it exerts a more strength electric force.

Next, you use the equation (1) to calculate the acceleration:

`a=(qE)/(m)=((1.6*10^(-19)C)(761.28N/C))/(9.1*10^(-31)kg)=1.33*10^(14)(m)/(s^2)`

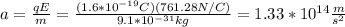
Next, you can calculate the time for which the particle is at 10.0 cm from the charge 1, by using the following formula:

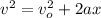`v^2=v_o^2+2ax` (3)

x: distance traveled by the electron = 0.17m - 0.10m = 0.07m

vo: initial speed of the electron = 0 m/s

You replace the values of the parameters in the equation (3):

`v=√(v_o^2+2ax)\\\\v=\sqrt{2(1.33*10^(14)m/s^2)(0.07m)}=4.31*10^6(m)/(s)`

The speed of the electron when it is at 0.10 m from the charge 1 is 4.31*10^6 m/s