Answer:
Point used by Harold was:
(7, 0)
Explanation:
Given that
Equation of linear function used by Harold:
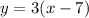
We know that linear equation in point slope form can be represented as:
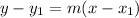
Where
 are the coordinates of a given point.
are the coordinates of a given point.
 is the slope of line.
is the slope of line.
Formula for Slope, m is given as:
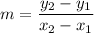
Where
 and
and
 are the two points on the line.
are the two points on the line.
If slope and a point with coordinates
 is know, the equation of a line can be represented in linear form as:
is know, the equation of a line can be represented in linear form as:
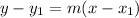 ....... (1)
....... (1)
Now, the given equation is:
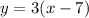
Re-writing the equation with a slight modification:
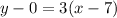
Now, comparing the above equation with equation (1):
We get that:
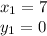
So, the point used by Harold is (7, 0).