Answer:
a) 21.58% probability that exactly 3 people are repeat offenders
b) 97.91% probability that at least one person is a repeat offender
c) 3.69
d) 1.83
Explanation:
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.

And p is the probability of X happening.
The expected value of the binomial distribution is:
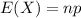
The standard deviation of the binomial distribution is:
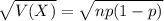
9% of all drivers arrested for DUI (Driving Under the Influence) are repeat offenders
This means that
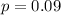
41 people arrested for DUI in Illinois are selected at random.
This means that
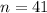
a. What is the probability that exactly 3 people are repeat offenders?
This is P(X = 3).

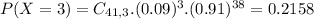
21.58% probability that exactly 3 people are repeat offenders
b. What is the probability that at least one person is a repeat offender?
Either none are repeat offenders, or at least one is. The sum of the probabilities of these outcomes is 1. So
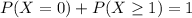
We want
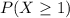 .
.
Then
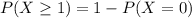
In which


97.91% probability that at least one person is a repeat offender
c. What is the mean number of repeat offenders?

d. What is the standard deviation of the number of repeat offenders?
