Answer:
The range of crying times within 68% of the data is (5.9, 8.1).
The range of crying times within 95% of the data is (4.8, 9.2).
The range of crying times within 99.7% of the data is (3.7, 10.3).
Explanation:
According to the Empirical Rule in a normal distribution with mean µ and standard deviation σ, nearly all the data will fall within 3 standard deviations of the mean. The empirical rule can be broken into three parts:
- 68% data falls within 1 standard deviation of the mean. That is P (µ - σ ≤ X ≤ µ + σ) = 0.68.
- 95% data falls within 2 standard deviations of the mean. That is P (µ - 2σ ≤ X ≤ µ + 2σ) = 0.95.
- 99.7% data falls within 3 standard deviations of the mean. That is P (µ - 3σ ≤ X ≤ µ + 3σ) = 0.997.
The mean and standard deviation are:
µ = 7
σ = 1.1
Compute the range of crying times within 68% of the data as follows:
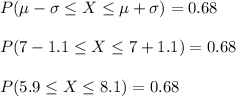
The range of crying times within 68% of the data is (5.9, 8.1).
Compute the range of crying times within 95% of the data as follows:

The range of crying times within 95% of the data is (4.8, 9.2).
Compute the range of crying times within 99.7% of the data as follows:
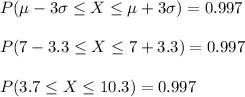
The range of crying times within 99.7% of the data is (3.7, 10.3).