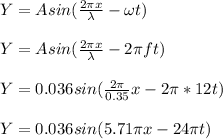Question

The tension in a string is 15 N, and its linear density is 0.85 kg/m. A wave on the string travels toward the -x direction; it has an amplitude of 3.6 cm and a frequency of 12 Hz. What are the:

a. Speed
b. Wavelength of the wave?
c. Write down a mathematical expression for the wave, substituting numbers for the variables

Answer 1

(a) The speed of the wave, v is 4.2 m/s

(b) Wavelength of the wave, λ is 0.35 m

(c) mathematical expression of the wave, Y = 0.036sin(5.71πx - 24πt)

Step-by-step explanation:

Given;

tension on the string, T = 15 N

Linear density, μ = 0.85 kg/m

amplitude of the wave, A = 3.6 cm = 0.036 m

frequency of the wave, f = 12 Hz

(a) The speed of the wave, v is calculated as;

`v = \sqrt{(T)/(\mu) } \\\\v = \sqrt{(15)/(0.85) }\\\\v = 4.2 \ m/s`

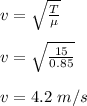
(b) Wavelength of the wave, λ

v = fλ

λ = v / f

λ = 4.2 / 12

λ = 0.35 m

(c) mathematical expression of the wave;

`Y = Asin((2\pi x)/(\lambda) -\omega t)\\\\Y = Asin((2\pi x)/(\lambda) -2\pi f t)\\\\Y = 0.036sin ((2\pi )/(0.35)x -2\pi *12 t)\\\\Y = 0.036sin (5.71 \pi x - 24 \pi t)`