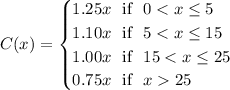Question

A supply store sells black oil sunflower seed using the following pricing: • The first 5 pounds costs $1.25 per pound • The next 10 pounds costs $1.10 per pound • The next 10 pounds costs $1.00 per pound • Any seed past the first 25 pounds costs $0.75 per pound what would the piecewise function C(x) for the cost of buying x pounds of black oil sunflower seed?

Answer 1

`C(x)=\begin{cases} 1.25x ~~\text{if}~~0<x\le5\\1.10x~~\text{if}~~5<x\le15\\1.00x~~\text{if}~~15<x\le25\\0.75x~~\text{if}~~x>25 \end{cases}`

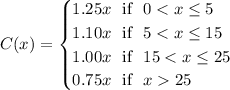
Explanation:

Let x represent the number of pounds of sunflower seed

Let Cₙ (x) represent the cost of buying x pound in the given interval n

Such that

`C(x)=\begin{cases} C_1(x) \\.\\.\\.\\C_n(x) \end{cases}`

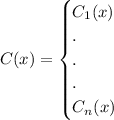
Let find different pieces and their conditions.

1st Part

For 1 to 5 pound, the rate is 1.25/pound

Which means total cost is 1.25x when x lies is from 1 to 5. Hence

C₁(x) = 1.25x if 0 < x ≤ 5

2nd Part

For greater than 5 to 15 pound, the rate is 1.10/pound

Which means total cost is 1.10x when x lies is from greater than 5 to 15. Hence

C₂(x) = 1.10x if 5 < x ≤ 15

3rd Part

For greater than 15 to 25 pound, the rate is 1.00/pound

Which means total cost is 1.00x when x lies is from greater than 15 to 25. Hence

C₃(x) = 1.00x if 15 < x ≤ 25

4rth Part

For greater 25 pound, the rate is 0.75/pound

Which means total cost is 0.75x when x lies is from greater than 25. Hence

C₄(x) = 0.75x if x>25

Piecewise Function:

Combine all to write the piecewise function

`C(x)=\begin{cases} 1.25x ~~\text{if}~~0<x\le5\\1.10x~~\text{if}~~5<x\le15\\1.00x~~\text{if}~~15<x\le25\\0.75x~~\text{if}~~x>25 \end{cases}`