Question

To decide whether two different types of steel have the same true average fracture toughness values, n specimens of each type are tested, yielding the following results.

Type Sample Average Sample SD
1 60.7 1.0
2 60.5 1.0

Required:
a. Calculate the P-value for the appropriate two-sample z test, assuming that the data was based on n = 100. (Round your answer to four decimal places.)
b. Calculate the P-value for the appropriate two-sample z test, assuming that the data was based on n = 500. (Round your answer to four decimal places.)
c. Is the small P-value for n = 500 indicative of a difference that has practical significance

Answer 1

a. P-value = 0.1589

b. P-value = 0.0016

Explanation:

a. This is a hypothesis test for the difference between populations means.

The claim is that the two types of steel have different true average fracture toughness values.

Then, the null and alternative hypothesis are:

`H_0: \mu_1-\mu_2=0\\\\H_a:\mu_1-\mu_2\\eq 0`

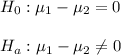
The significance level is α=0.05.

The sample 1, of size n1=100 has a mean of 60.7 and a standard deviation of 1. The sample 2, of size n2=100 has a mean of 60.5 and a standard deviation of 1.

The difference between sample means is Md=0.2.

`M_d=M_1-M_2=60.7-60.5=0.2`

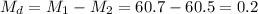
The estimated standard error of the difference between means is computed using the formula:

`s_(M_d)=\sqrt{(\sigma_1^2+\sigma_2^2)/(n)}=\sqrt{(1^2+1^2)/(100)}\\\\\\s_(M_d)=\sqrt{(2)/(100)}=√(0.02)=0.1414`

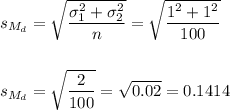
Then, we can calculate the t-statistic as:

`t=(M_d-(\mu_1-\mu_2))/(s_(M_d))=(0.2-0)/(0.1414)=(0.2)/(0.1414)=1.4142`

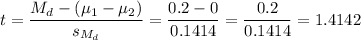
The degrees of freedom for this test are:

`df=n_1+n_2-2=100+100-2=198`

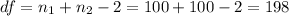
This test is a two-tailed test, with 198 degrees of freedom and t=1.4142, so the P-value for this test is calculated as (using a t-table):

`P-value=2\cdot P(t>1.4142)=0.1589`

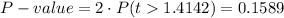
As the P-value (0.1589) is greater than the significance level (0.05), the effect is not significant.

The null hypothesis failed to be rejected.

There is not enough evidence to support the claim that the two types of steel have different true average fracture toughness values.

b. As the sample size changes, the standard error and the degress of freedom change.

The estimated standard error of the difference between means is computed using the formula:

`s_(M_d)=\sqrt{(\sigma_1^2+\sigma_2^2)/(n)}=\sqrt{(1^2+1^2)/(500)}\\\\\\s_(M_d)=\sqrt{(2)/(500)}=√(0.004)=0.0632`

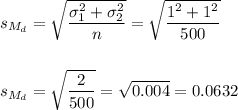
Then, we can calculate the t-statistic as:

`t=(M_d-(\mu_1-\mu_2))/(s_(M_d))=(0.2-0)/(0.0632)=(0.2)/(0.0632)=3.1623`

The degrees of freedom for this test are:

`df=n_1+n_2-2=500+500-2=998`

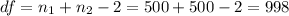
This test is a two-tailed test, with 998 degrees of freedom and t=3.1623, so the P-value for this test is calculated as (using a t-table):

`P-value=2\cdot P(t>3.1623)=0.0016`

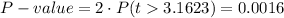
As the P-value (0.0016) is smaller than the significance level (0.05), the effect is significant.

The null hypothesis is rejected.

There is enough evidence to support the claim that the two types of steel have different true average fracture toughness values.