Question

A population of monkeys' tail lengths is normally distributed with a mean of 25 cm with a standard deviation of 8 cm. I am preparing to take a sample of size 256 from this population, and record the tail length of each monkey in my sample. What is the probability that the mean of my sample will be between 24 and 25 cm?

Answer 1

The probability that the mean of my sample will be between 24 and 25 cm

P(24 ≤X⁻≤25) = 0.4772

Explanation:

Step(i):-

Given mean of the Population 'μ'= 25c.m

Given standard deviation of the Population 'σ' = 8c.m

Given sample size 'n' = 256

Let X₁ = 24

`Z_(1) = (x_(1)-mean )/((S.D)/(√(n) ) ) = (24-25)/((8)/(√(256) ) ) = -2`

Let X₂ = 25

`Z_(2) = (x_(2)-mean )/((S.D)/(√(n) ) ) = (25-25)/((8)/(√(256) ) ) = 0`

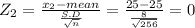
Step(ii):-

The probability that the mean of my sample will be between 24 and 25 cm

P(24 ≤X⁻≤25) = P(-2≤ Z ≤0)

= P( Z≤0) - P(Z≤-2)

= 0.5 + A(0) - (0.5- A(-2))

= A(0) + A(2) ( ∵A(-2) =A(2)

= 0.000+ 0.4772

= 0.4772

Final answer:-

The probability that the mean of my sample will be between 24 and 25 cm

P(24 ≤X⁻≤25) = 0.4772