Answer:
The angular acceleration of the wheel of fortune is -0.125 radians per square second.
Step-by-step explanation:
Let suppose that wheel of fortune is decelerated at constant rate, given that wheel of fortune stops after rotating 540 degrees with an initial tangential velocity of 2 meters per second, the initial angular velocity and the kinematic expression of final angular speed as a function of angular acceleration and position are, respectively:


Where:
 - Initial tangential velocity, measured in meters per second.
- Initial tangential velocity, measured in meters per second.
 - Radius of the wheel of fortune, measured in meters.
- Radius of the wheel of fortune, measured in meters.
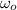 - Initial angular velocity, measured in radians per second.
- Initial angular velocity, measured in radians per second.
 - Final angular velocity, measured in radians per second.
- Final angular velocity, measured in radians per second.
 - Angular acceleration, measured in radians per square second.
- Angular acceleration, measured in radians per square second.
 - Final angular position, measured in radians.
- Final angular position, measured in radians.
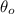 - Initial angular position, measured in radians.
- Initial angular position, measured in radians.
Angular acceleration is now cleared in the second expression:
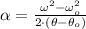
Given that
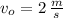 and
and
 , the initial angular velocity is:
, the initial angular velocity is:
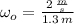

Now, if
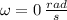 ,
,
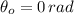 and
and
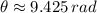 (180° = π rad), the angular acceleration of the wheel is:
(180° = π rad), the angular acceleration of the wheel is:
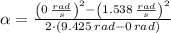
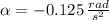
The angular acceleration of the wheel of fortune is -0.125 radians per square second.