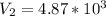Question

A balloon filled with helium has a volume of 4.5 × 103 L at 25°C. What volume will the balloon occupy at 50°C if the pressure surrounding the balloon remains constant?

Answer 1

`V_2 = 4.87 * 10^3`

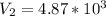
Step-by-step explanation:

This question is an illustration of ideal Gas Law;

The given parameters are as follows;

Initial Temperature = 25C

Initial Volume = 4.5 * 10³L

Required

Calculate the volume when temperature is 50C

NB: Pressure remains constant;

Ideal Gas Law states that;

`PV = nRT`

The question states that the pressure is constant; this implies that the constant in the above formula are P, R and n

Divide both sides by PT

`(PV)/(PT) = (nRT)/(PT)`

`(V)/(T) = (nR)/(P)`

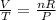
Represent
`(nR)/(P)` with k

`(V)/(T) = k`

`k = (V_1)/(T_1) = (V_2)/(T_2)`

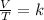
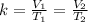
At this point, we can solve for the required parameter using the following;

`(V_1)/(T_1) = (V_2)/(T_2)`

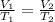
Where V1 and V2 represent the initial & final volume and T1 and T2 represent the initial and final temperature;

From the given parameters;

V1 = 4.5 * 10³L

T1 = 25C

T2 = 50C

Convert temperatures to degree kelvin

V1 = 4.5 * 10³L

T1 = 25 +273 = 298K

T2 = 50 + 273 = 323K

Substitute values for V1, T1 and T2 in
`(V_1)/(T_1) = (V_2)/(T_2)`

`(4.5 * 10^3)/(298) = (V_2)/(323)`

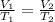
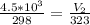
Multiply both sides by 323

`323 * (4.5 * 10^3)/(298) = (V_2)/(323) * 323`

`323 * (4.5 * 10^3)/(298) = V_2`

`V_2 = 323 * (4.5 * 10^3)/(298)`

`V_2 = (323 * 4.5 * 10^3)/(298)`

`V_2 = (1453.5 * 10^3)/(298)`

`V_2 = 4.87 * 10^3`

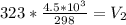
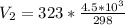
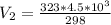
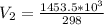
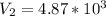
Hence, the final volume at 50C is
`V_2 = 4.87 * 10^3`