Question

A charged particle is observed traveling in a circular path in a uniform magnetic field. If the particle had been traveling four times as fast, the radius of the circular path would be:

Answer 1

The new radius of the trajectory of the particle is four times the previous radius

Step-by-step explanation:

In order to know what is the radius of the trajectory of the charged particle, if its speed is four times as fast, you take into account the following formula, which describes the radius of a charged particle in a magnetic field:

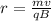`r=(mv)/(qB)` (1)

If the speed of the particle is for time as fast, that is, v' = 4v, you obtain, in the equation (1):

`r'=(mv')/(qB)=(m(4v))/(qB)=4(mv)/(qB)=4r`

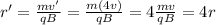
The new radius of the trajectory of the particle is four times the previous radius