Question

A chemist dissolves 867. mg of pure barium hydroxide in enough water to make up 170. mL of solution. Calculate the pH of the solution. (The temperature of the solution is 25 degree C.) Be sure your answer has the correct number of significant digits.

Answer 1

Answer: The pH of the solution is 11.2

Step-by-step explanation:

Molarity of a solution is defined as the number of moles of solute dissolved per liter of the solution.

`Molarity=(n* 1000)/(V_s)`

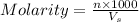
where,

n = moles of solute

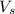`V_s` = volume of solution in ml

moles of
`Ba(OH)_2` =
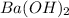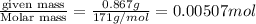`\frac{\text {given mass}}{\text {Molar mass}}=(0.867g)/(171g/mol)=0.00507mol` (1g=1000mg)

Now put all the given values in the formula of molality, we get

`Molarity=(0.00507* 1000)/(170)`

`Molarity=0.0298`

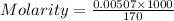
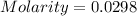
pH or pOH is the measure of acidity or alkalinity of a solution.

pH is calculated by taking negative logarithm of hydrogen ion concentration.

`pOH=-\log [OH^-]`

`Ba(OH)_2\rightarrow Ba^(2+)+2OH^(-)`

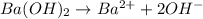
According to stoichiometry,

1 mole of
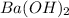`Ba(OH)_2` gives 2 mole of
`OH^-`

Thus 0.0298 moles of
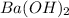`Ba(OH)_2` gives =
`(2)/(1)* 0.0298=0.0596` moles of
`OH^-`

Putting in the values:

`pOH=-\log[0.0596]=2.82`

`pH+pOH=14`

`pH=14-2.82`

`pH=11.2`

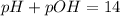
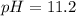
Thus the pH of the solution is 11.2