Question

A car traveling with velocity v is decelerated by a constant acceleration of magnitude a. It takes a time t to come to rest. If its initial velocity were doubled, the time required to stop would

Answer 1

If the initial speed is doubled the time is also doubled

Step-by-step explanation:

You have that a car with velocity v is decelerated by a constant acceleration a in a time t.

You use the following equation to establish the previous situation:

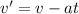`v'=v-at` (1)

v': final speed of the car = 0m/s

v: initial speed of the car

From the equation (1) you solve for t and obtain:

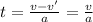`t=(v-v')/(a)=(v)/(a)` (2)

To find the new time that car takesto stop with the new initial velocity you use again the equation (1), as follow:

`v'=v_1-at'` (3)

v' = 0m/s

v1: new initial speed = 2v

t': new time

You solve the equation (3) for t':

`0=2v-at'\\\\t'=(2v)/(a)=2t`

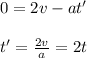
If the initial speed is doubled the time is also doubled