Question

You attach a 1.70 kg block to a horizontal spring that is fixed at one end. You pull the block until the spring is stretched by 0.200 m and release it from rest. Assume the block slides on a horizontal surface with negligible friction. The block reaches a speed of zero again 0.200 s after release (for the first time after release). What is the maximum speed of the block (in m/s)

Answer 1

maximum speed of the block is 3.14 m/s

Step-by-step explanation:

given data

mass = 1.70 kg

stretch in the spring = 0.2 m

time take by block to come to zero t = 0.2 s

solution

we know that Time period of oscillation (T) that is express as

T = 2t ......................1

put here value

T = 2 (0.2)

T = 0.4 s

so here time period is express as

T =
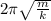`2\pi \sqrt{(m)/(k)}` ................2

here k is spring constant of the spring so put here value

0.4 =
`2(\pi ) \sqrt{(1.70)/(k)}`

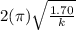
here k will be

k = 419.02 N/m

so we use here conservation of energy that is

Maximum kinetic energy = Maximum spring potential energy ............3

(0.5) m v² = (0.5) k x²

here v is maximum speed block

so put here value and we get

(1.70) v² = (419.02) (0.2)²

v = 3.14 m/s

so maximum speed of the block is 3.14 m/s