Question

The owner of a manufacturing plant employs eighty people. As part of their personnel file, she asked each one to record to the nearest one-tenth of a mile the distance they travel one way from home to work. The distances for a random sample of six employees are listed below: 26 32 29 16 45 19 Find the variance for the given data.

Answer 1

The variance of the given data is 106.9667

Explanation:

The variance, S² of a sample is given by the expression;

`S^2 = \frac{\sum \left (x_(i)-\bar{x} \right )^(2)}{n-1}`

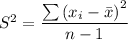
Where:

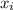`x_(i)` = One of the observations

`\bar{x}` = The mean of the observations

n = Number in the sample = 6

The mean = Σ
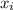`x_(i)`/n = (26 + 32 + 29 + 16 + 45 + 19 )/6 = 167/6 = 27.833

Therefore, we have;

S² = ((26 - 27.833)²+(32 - 27.833)²+(29 - 27.833)²+(16 - 27.833)²+(45 - 27.833)²+(19 - 27.833)²)/(6 - 1) = 106.9667.