Answer:

Explanation:
Given
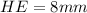
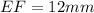
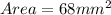
Find the length of HG
First, it should be noted that the displayed figure is a trapezium
The area of a trapezium is calculated by multiplying the sum of parallel sides by half its height;
In this case;

Substitute the values of Area, HE and EF
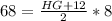
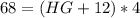
Divide both sides by 4
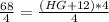
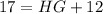
Subtract 12 from both sides
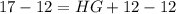


Hence, the length of HG is 5mm