Question

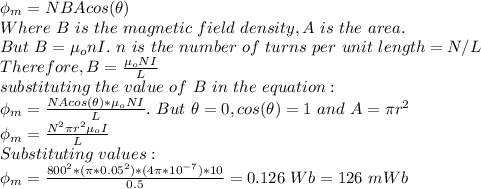
A solenoid 50-cm long with a radius of 5.0 cm has 800 turns. You find that it carries a current of 10 A. The magnetic flux through it is approximately Group of answer choices

Answer 1

126 mWb

Step-by-step explanation:

Given that:

length (L) = 50 cm = 0.5 m, radius (r) = 5 cm = 0.05 m, current (I) = 10 A, number of turns (N) = 800 turns.

We assume that the magnetic field in the solenoid is constant.

The magnetic flux is given as:

`\phi_m=NBAcos(\theta)\\Where\ B\ is\ the\ magnetic\ field\ density,A\ is \ the\ area.\\But\ B =\mu_onI.\ n \ is\ the\ number\ of\ turns\ per\ unit \ length=N/L\\Therefore,B=(\mu_oNI)/(L) \\substituting\ the\ value\ of\ B\ in\ the\ equation: \\\phi_m=(NAcos(\theta)*\mu_oNI)/(L) .\ But \ \theta=0,cos(\theta)=1\ and\ A=\pi r^2\\ \phi_m=(N^2\pi r^2\mu_oI)/(L) \\Substituting\ values:\\\phi_m=(800^2*(\pi*0.05^2)*(4\pi*10^(-7))*10)/(0.5)=0.126\ Wb=126\ mWb`