The commute time for people in a city has an exponential distribution with an average of 0.5 hours. What is the probability that a randomly selected person in this city will hav…

Question

asked Jun 4, 2021 234k views

1 Answer

Jeta · Answer 1 · 2021-06-09T06:19:43+0000

Answer:

0.314 = 31.4% probability that a randomly selected person in this city will have a commute time between 0.4 and 1 hours

Explanation:

Exponential distribution:

The exponential probability distribution, with mean m, is described by the following equation:

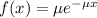
$f(x) = \mu e^(-\mu x)$

In which
$\mu = (1)/(m)$ is the decay parameter.

The probability that x is lower or equal to a is given by:

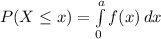
$P(X \leq x) = \int\limits^a_0 {f(x)} \, dx$

Which has the following solution:

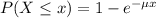
$P(X \leq x) = 1 - e^(-\mu x)$

The probability of finding a value higher than x is:

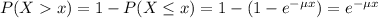
$P(X > x) = 1 - P(X \leq x) = 1 - (1 - e^(-\mu x)) = e^(-\mu x)$

In this question:

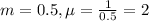
$m = 0.5, \mu = (1)/(0.5) = 2$

What is the probability that a randomly selected person in this city will have a commute time between 0.4 and 1 hours?

$P(0.4 \leq X \leq 1) = P(X \leq 1) - P(X \leq 0.4)$

In which

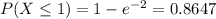
$P(X \leq 1) = 1 - e^(-2) = 0.8647$

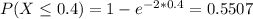
$P(X \leq 0.4) = 1 - e^(-2*0.4) = 0.5507$

So

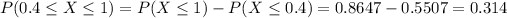
$P(0.4 \leq X \leq 1) = P(X \leq 1) - P(X \leq 0.4) = 0.8647 - 0.5507 = 0.314$

0.314 = 31.4% probability that a randomly selected person in this city will have a commute time between 0.4 and 1 hours