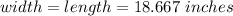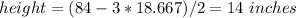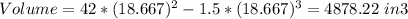Answer:
Dimensions: 18.667 x 18.667 x 14 inches
Volume: 4878.22 in3
Explanation:
If the length plus the girth does not exceed 84 inches, we have:
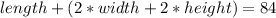
The box has a square base, to length = width, then we have:
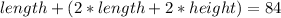
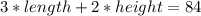
The volume of the box is given by the equation:
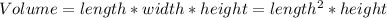
From the first equation, we have:

Using this height in the volume equation, we have:

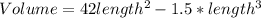
To find the maximum volume, we can find the value of length that makes the derivative of the volume in relation to the length equal zero:
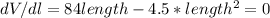
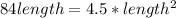
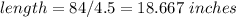
So the width, the height and the volume of the package are: