Answer:
1. B. False
2. B. False
3. A. True
4. B. False
5. A. True
6. A. True
7. A. True
Step-by-step explanation:
1. B. False
The relation of Reynolds' number, Reₓ to boundary layer thickness δ at a point x is given by the relation
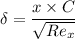
That is the boundary layer thickness is inversely proportional to the square root of the Reynolds' number so that if the Reynolds' number were to increase, the boundary layer thickness would decrease
Therefore, the correct option is B. False
2. B. False
From the relation

As the outer flow velocity increases, the boundary layer thickness diminishes
3. A. True
As the viscous force is increased the boundary layer thickness increases
4. B. False
Boundary layer thickness is inversely proportional to velocity
5. A. True
The boundary layer model developed by Ludwig Prandtl is a special case of the Navier-Stokes equation
6. A. True
Given a definite boundary layer thickness, the curve representing the boundary layer thickness is a streamline
7. A. True
The boundary layer approximation by Prandtl Euler bridges the gap between the Euler (slip boundary conditions) and Navier-Stokes (no slip boundary conditions) equations.