Given Information:
Mean = μ = 22
Standard deviation = σ = 5
Required Information:
1. z-value = ?
2. P(22 < X < 25) = ?
3. P(X < 18) = ?
Answer:
1. z-value = 0.6
2. P(22 < X < 25) = 22.58%
3. P(X < 18) = 21.19%
Explanation:
What is Normal Distribution?
Normal Distribution is a continuous probability distribution and is symmetrical around the mean. The shape of this distribution is like a bell curve and most of the data is clustered around the mean. The area under this bell shaped curve represents the probability
1. We want to find out the z-value associated with 25
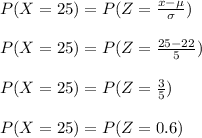
Therefore, the z-value associated with X = 25 is 0.6
2. We want to find out the proportion of the population that is between 22 and 25.
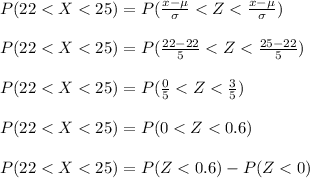
The z-score corresponding to 0 is 0.50
The z-score corresponding to 0.6 is 0.7258
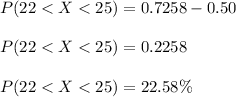
Therefore, the proportion of the population that is between 22 and 25 is 22.58%
3. We want to find out the proportion of the population that is less than 18
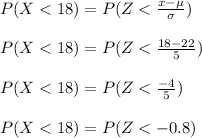
The z-score corresponding to -0.8 is 0.2119
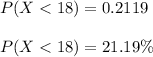
Therefore, the proportion of the population that is less than 18 is 21.19%
How to use z-table?
Step 1:
In the z-table, find the two-digit number on the left side corresponding to your z-score. (e.g 1.0, 2.2, 0.5 etc.)
Step 2:
Then look up at the top of z-table to find the remaining decimal point in the range of 0.00 to 0.09. (e.g. if you are looking for 0.6 then go for 0.00 column)
Step 3:
Finally, find the corresponding probability from the z-table at the intersection of step 1 and step 2.