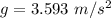Question

While on Mars, two astronauts repeat the pendulum experiment you conducted earlier this term in your physics lab. They go off script and plot the pendulum length vs. the square of the period. Their best fit is a line with y = 0.091 x and R^2=1.

Recall that the theoretical period of a pendulum is T = 2pi(L/g)^1/2. Apply your understanding of mathematical modeling to determine what the constant 0.091 in the astronauts' equation of the best fit line is equal to in this case and use that to find their experimental value of g on Mars in m/s^2.

Answer 1

The constant 0.091 in the astronauts' equation of the best fit line is equal to
`(L)/(T^2)`

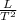
The value of g on Mars is
`g = 3.593 \ m/s^2`

Step-by-step explanation:

From the question we are told that

The line of best fit is defined by the equation
`y = 0.091 x \ and \ R^2 = 1`

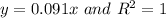
Now the equation of a straight line is defined as

`y = mx + c`

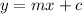
Now comparing the given equation to this we have that

`m = slope = 0.091`

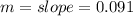
Now from the graph the formula for the slope is

`m = (L)/(T^2)`

=>
`0.091 = (L)/(T^2)`

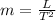
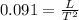
Now from the question we are told that

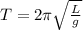
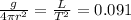
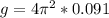
`T = 2 \pi \sqrt{(L)/(g) }`

=>
`(g)/(4\pi r^2) = (L)/(T^2) = 0.091`

=>
`g = 4\pi^2 * 0.091`

=>
`g = 3.593 \ m/s^2`